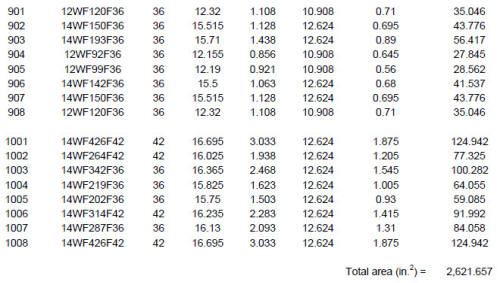
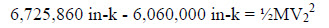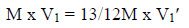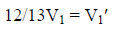The Missing Jolt: A Simple Refutation of the NIST-Bazant Collapse Hypothesis
by Graeme MacQueen & Tony Szamboti
Journal of 911 Studies, Volume 24
1 January 2009
April 22, 2009*
NOTICE: THIS WORK MAY BE PROTECTED BY COPYRIGHT
YOU ARE REQUIRED TO READ THE COPYRIGHT NOTICE AT THIS LINK BEFORE YOU READ THE FOLLOWING WORK, THAT IS AVAILABLE SOLELY FOR PRIVATE STUDY, SCHOLARSHIP OR RESEARCH PURSUANT TO 17 U.S.C. SECTION 107 AND 108. IN THE EVENT THAT THE LIBRARY DETERMINES THAT UNLAWFUL COPYING OF THIS WORK HAS OCCURRED, THE LIBRARY HAS THE RIGHT TO BLOCK THE I.P. ADDRESS AT WHICH THE UNLAWFUL COPYING APPEARED TO HAVE OCCURRED. THANK YOU FOR RESPECTING THE RIGHTS OF COPYRIGHT OWNERS.
In its Final Report on the Collapse of the World Trade Center Towers, the National Institute of Standards and Technology summarizes its three year study and outlines its explanation of the total collapse of WTC 1 and WTC 2. [1]
Readers of the report will find that the roughly $20 million expended on this effort have resulted in an explanation of the total collapse of these buildings that is so vague it barely qualifies as a hypothesis. But it does have one crucial feature of a hypothesis: it is, in principle, falsifiable. In fact, it is easy to demonstrate that it is false.
In this paper we will, concentrating on the North Tower, offer a refutation that is:
• easy to understand but reasonably precise
• capable of being stated briefly
• verifiable by any reader with average computer skills and a grasp of simple mathematics.
NIST’s Hypothesis of Total Collapse:
Three essential elements of NIST’s hypothesis of total collapse are made explicit in the Final Report and the companion volumes of the study:
1. Because of damage to stories 93 to 98, and especially because of column buckling due to fire, the top 12 stories of the North Tower (99-110) plus the roof were, in effect, separated from the rest of the Tower and began to behave as a unit. [2]
2. This “rigid block” of 12 stories plus the roof began to move. First it tilted, and then it abruptly fell onto the stories beneath it. [3]
3. The fall of the rigid block caused such damage to the lower structure that “global collapse began.”[4]
The rigidity of the upper block of stories is crucial to this explanation. If the upper block were to break, disintegrate or flow on impact it would certainly not threaten the 92 intact floors beneath it.
In addition, the rigid block had to fall onto the rest of the building. Although this seems obvious, the NIST authors are often shy about saying it. We hear about the rigid block’s “descent.”[5] We hear of tilting and “downward movement.”[6] We have to look carefully to find the NIST authors using the language of falling. Whatever the reasons for their reticence, it is clear that it will not do for the upper block to ease itself onto the building beneath it, with a gradual creaking of buckled columns and sagging floors. If this were to happen, why would the structure beneath collapse?
There was nothing special about the weight of the upper block, rigid or otherwise. The lower part of the Tower had held up this weight without difficulty since 1970. The lower block had 283 cold steel columns, with less than 30% of their total load capacity being utilized for gravity loads, because of the factors of safety designed into the structure and the need to withstand high winds—and gravity loads were essentially the only loads the columns would have been subject to on a day such as 9/11 with little wind. The lower block was not weak, nor (excluding stories 93-98) was it damaged by plane impact or fire. The weight of the upper block posed no threat to it. If there were to be a threat, it had to come from the momentum of the upper block. But momentum is a product of mass and velocity, and since the upper block could not increase its mass it had to increase, if it were to become a threat, its velocity. Since NIST’s theory assumes the only energy at play at this stage of events was gravitational, the upper block had to fall, and the greater its velocity the greater its momentum. The longer and the less impeded its fall, the greater would be its impact on the lower structure. So it is no surprise that the NIST authors, however shy they are about affirming it, eventually come out in favour of the falling of the upper block. [7]
Zdenek Bazant and Yong Zhou, with whose September 13, 2001 back-of-the-envelope theory (with subsequent revisions and additions) NIST largely agrees, have never hesitated to say that the upper block fell. [8] Bazant has likewise been frank about the need for severe impact as the upper and lower structures met: he believes the impact may have been powerful enough to have been recorded by seismometers. [9] In his view, collapse initiation of the lower structure required “one powerful jolt.”[10] Of course, if there was a powerful jolt to the lower structure there must also have been a powerful jolt to the upper falling structure, in accord with Newton’s Third Law.
In order to keep a sense of reality as we discuss NIST’s theory it may be useful to label the three interacting parts of the North Tower, as they are pictured by NIST, as RB-12+, DS-6 and RB-92. Where RB stands for rigid block, DS stands for damaged structure, and the numbers following the letters refer to the number of stories in each structure. The upper block comprised the 12 stories of 99-110 as well as the roof structure with antenna and hat truss; the intermediate area was damaged by plane impact and fire and was six stories high (93-98 inclusive); and the lower block was rigid and comprised, in addition to subterranean levels, the first 92 stories of the building.
These designations actually underestimate the contrast between RB-12+ and RB-92, because the latter was not only largely undamaged by fire but was more massive per story. It was also stronger: the Tower’s columns tapered as they ascended. [11] Yet the fall of RB-12+, we are supposed to believe, put a catastrophic end to DS-6 and RB-92.
What NIST essentially says, agreeing with Bazant, is that the lighter and weaker part initially fell with a powerful jolt onto the heavier and stronger part, which could not withstand its momentum, and that this caused a progressive collapse to initiate smashing the lower block to bits all the way to the ground.
The NIST Final Report does not tell us what happened to RB-12+ after its impact with the two structures beneath it. Did it fall through them all the way to the ground (that is, to the rubble heap on the ground), maintaining considerable mass and rigidity the whole time--as Bazant argued in 2001 and has continued to argue? [12]
On this the NIST authors are silent.
NIST also does not tell us how far RB-12+ fell before its impact with intact structure. Did it fall one story (roughly 12 feet), or several stories? We are left in the dark. Once again Bazant comes to the rescue. It fell “at least one story,” he says. [13]
To his credit, Bazant is willing to state the essential elements of the hypothesis. If this hypothesis is to hold any water at all there must be substantial impact: RB-12+ has a lot of work to do, so it had better fall at least one story.
As we will show, for the purposes of the present refutation it does not matter whether RB-12+ fell one story, six stories, or somewhere in between.
The Necessary Jolt:
As Bazant has said, when the top part fell and struck the stories beneath it, there had to be a powerful jolt. While a jolt entails acceleration of the impacted object it requires deceleration of the impacting object. Even a hammer hitting a nail decelerates, and if the hammer is striking a strong, rigid body fixed to the earth its deceleration will be abrupt and dramatic.
Although NIST does not explicitly speak, like Bazant, of a “jolt”, and may therefore be thought to evade this paper’s refutation, it is impossible for NIST to escape the implications of its own assertions. The NIST report speaks of a strong, rigid structure (the upper structure or rigid block) falling freely onto another strong, rigid structure (the intact part of the building below the damaged area): the jolt cannot be avoided. [14]
This was a necessary jolt. Without it the required work could not have been done.
Testing for Deceleration:
If a jolt occurred there would have been high short-term deceleration of the upper block. Why not simply check for this deceleration? It is not difficult. We will:
• examine a video clip of the North Tower’s collapse
• find a point on the upper block of the North Tower, the progress of which can be observed and measured in the early stages of the collapse
• plot the progress of this point on a graph
• check for evidence of deceleration
We have chosen a well known video clip of the collapse associated with French film maker, Etienne Sauret. [15] The Sauret clip has advantages over many others. It is a single, continuous sequence with no changes in camera angle and no zooming in and out. There is a very slight shift in the camera position relative to distant objects caused by a trembling of the camera several seconds prior to the collapse, but this is irrelevant to us since all our measurements are taken after the shift. The camera is very steady throughout the time we are making our measurements, as we can confirm by measuring the position of the picture frame relative to stationary objects. In addition, the image of the north face of the North Tower is exceptionally clear in these images.
Here is how we proceed: [16]
1. We save the Sauret footage to our hard drive.
2. We break the 1 minute, 56.53 second clip into 3497 equal segments or “frames.” Each frame is approximately 0.033 seconds in length (33 thousandths of a second).
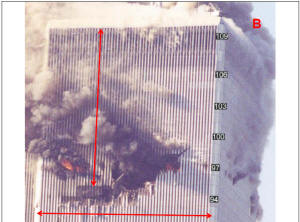
3. We find two points associated with the roof of the upper block of the North Tower whose progress we can measure. Two points are necessary since neither one is consistently visible but one of the two is always visible. The point whose fall we shall use in our computations is at the tip of a white device on the roof. (The distance between this point and the upper frame is called Distance A in Figure 1 below.) The other point is located at the interface of the upper white section of the roof and the lower dark section. (The distance between this point and the upper frame is called Distance B in Figure 1.) The difference between Distance B and Distance A is approximately 28 pixels. Where the white device on the upper right-hand corner of the roof is obscured by smoke, measurements of the roof interface have been taken and the position of the device has been obtained by subtracting 28.
4. We choose a set of frames that stretches from Frame 929, before the discernible beginning of the roof’s fall, to the last frame in which our point can be recognized before it disappears into the dust cloud, Frame 1024.
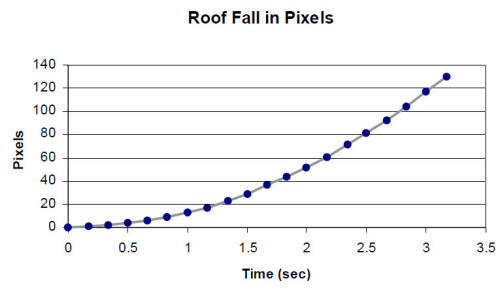
5. We measure the number of pixels separating the white device from the fixed upper edge of the video frame, computing the position of the device when necessary by measuring the position of the roof interface. We take one measurement at each five frames in the progress of the Tower’s collapse, ending up with 20 points.
6. Our measurement stretches from 30.93 seconds into the clip to 34.1 seconds into the clip, giving us a total interval of 3.17 seconds.
7. We find that during this interval the white device on the roof has fallen a distance represented by 130 pixels.
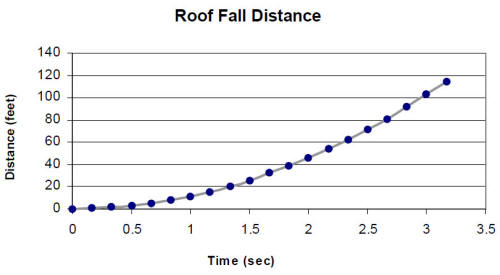
8. In order to get an approximation of the real distances at issue; we find a known vertical distance on the north face of the North Tower. (The Tower’s proportions have been distorted as it has been rendered into frame-by-frame format. See Appendix A for a description of our method of determining the known vertical distance and the ratio of pixels to feet.) We discover that in our frame-by-frame version of the Sauret video 1 pixel = 0.88 feet. We now know that the point on the roof has fallen approximately 114.4 feet. The figure is not precise -- there are the effects of foreshortening to consider (the roof and device are higher than the camera and the upper block, as it moves downward, tilts away from us) -- but the figures are close enough for our purpose because we are looking for changes in acceleration over time, not exact velocity values.
Figure 1: Sauret Video: Representative Frame with Key Points for Measuring the Roof’s Fall

9. We know that
d = ½ × g × t2
where d stands for distance, g stands for acceleration due to gravity, which is 32.174 ft./s2 at sea level, and t stands for time. Using this formula, we discover that a freely falling object would travel 161.6 feet in the time it took the roof to drop 114.4 feet.
10. We create two graphs. In the first the roof’s descent is given in pixels. In the second the roof’s fall is given in feet.
Figure 2: The Roof Fall: Pixels
Data:
Figure 3: The Roof Fall: Distance
Data:

Knowing the distance the roof fell, in equal time intervals, from our measurements, we can now determine its actual velocity, at each measured point through its fall, using symmetric differencing. The equation is
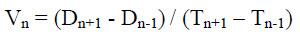
Vn = (Dn+1 - Dn-1) / (Tn+1 – Tn-1)
where V = velocity
D = distance
T = time
n = point in question
Data:
It may be noticed that the last point measured at 3.167 seconds is left off of the data table above and the velocity graph below. The reason for this is that each point the velocity is found for needs to have a point ahead of it as well as behind it, so this method cannot calculate the velocity for the last point measured. As it is known that the measurements were taken every five frames with a 30 frame per second video, the actual time can be resolved fairly precisely. The use of four places for time increments, of 0.1667 seconds between measurements, in the velocity calculation above, is done for accuracy.
Below is a graph of the actual velocity of the roof at each measurement point over the same time frame in which the distance was measured.
Figure 4: The Roof Velocity
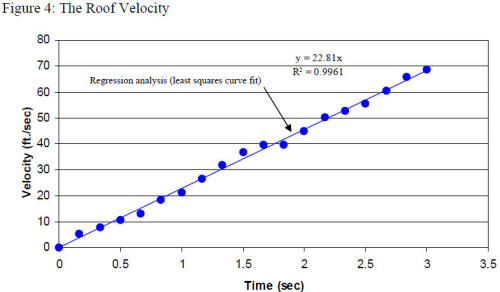
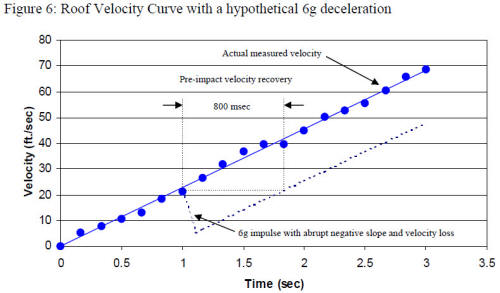
The velocity of the roof increases in a relatively linear way and is 68.65 ft./s after 3.00 seconds, which is about 71% of the free fall velocity of 96.52 ft./s for this fall time. At the actual measured distances and calculated velocities, the initial fall through one story would have taken place in approximately 1.0 second.
If the upper block, RB-12+, were rigid, as Bazant and NIST claim, the powerful jolt, required by Bazant to generate an impulsive load and explain the collapses of the Twin Towers, would show itself as an abrupt negative deviation in the otherwise positively sloped and virtually linear velocity graph.
For readers unfamiliar with the concept of an impulsive load, the impulse-momentum change equation is shown below and essentially shows that the change in momentum with respect to time provides the force involved in a collision.
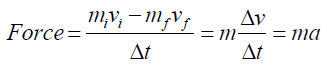
As stated earlier, it is only the velocity that changes with respect to the duration of the impulse, as the mass of an object is constant at all times everywhere in the universe. A change in velocity with respect to time is defined as either an acceleration or deceleration, depending on whether it is positive or negative. This acceleration or deceleration is then multiplied by the mass of the impacting object and provides the force involved in the collision, so the impulse equation ultimately reduces to the well known relation F = ma.
It is useful to refer to accelerations and decelerations in terms of the acceleration due to gravity, which is defined as 1g. The static weight of any item on earth is measured as the force due to the mass of the item multiplied by the acceleration of earth’s gravitational pull or 1g. An acceleration or deceleration of 1g is equal to 32.174 ft./s2, so if the deceleration of an impacting object during a collision is greater than this then the weight or force applied by the impacting object is amplified. To find the number of g’s involved one merely needs to divide the actual deceleration by 32.174 ft./s2.
Bazant claims that a minimum force amplification of 31g, or 31 times the static weight of the upper stories, could have occurred in a collision between the upper and lower blocks of the Twin Towers after a fall of one story. [17] With the 98th story columns completely collapsing, a distance between floor slabs of approximately 11.44 feet, and the actual measured velocity of 22.81 ft./s of the upper block at this point, the first collision would have occurred approximately one second into the fall. Regardless of the actual amplification, any impulse at the impact zone between the 98th and 99th story floor slabs capable of causing collapse continuation would have had to cause the columns on at least the first stories on either side of the impact to deform elastically, and plastically, and then to buckle. The deformations and buckling of the columns of the impacting stories, on both the lower and upper blocks, would cause a kinetic energy drain, which would reduce the velocity of the rigidly attached falling mass above them. Using energy methods we have calculated what effect these energy drains would have on the velocity of the upper block. Since the upper block would pick up the mass of the 98th floor in the impact there would also be a conservation of momentum component to the velocity reduction. From Appendices D and E we find the reduced velocity (Vreduced) of the upper block, after impact, considering the three energy drains and conservation of momentum, and it is
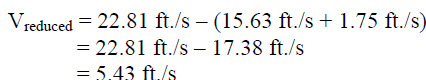
Vreduced = 22.81 ft./s – (15.63 ft./s + 1.75 ft./s)
= 22.81 ft./s – 17.38 ft./s
= 5.43 ft./s
Since the roof was part of the rigid upper block it would have displayed this momentary abrupt change in its velocity, from 22.81 ft./s to 5.43 ft./s, if the collapse were a natural occurrence.It should also be noted that the energy losses and conservation of momentum we have calculated and used here, to determine the velocity loss, are a minimum. We do not consider energy losses due to vibration of the building, heat, and sound, during the initiating impulse, all of which would have required energy from the impulse to produce and thus have an additional effect on velocity loss. The intent here is only to show that, even with a quantifiable minimum energy loss and conservation of momentum, the velocity loss would be quite dramatic, and should have been readily observed if an impulse capable of causing collapse had indeed occurred.
The graph below shows what the upper block velocity change would look like if a 31g impulse had occurred one story into the fall, with its velocity at least momentarily reduced in a significant way after impact.
Figure 5: Roof Velocity Curve with a hypothetical 31g deceleration
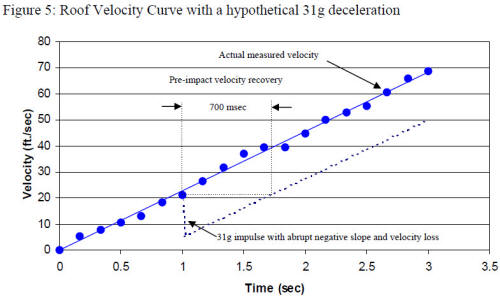
The fact that a 31g impulse requires a deceleration of 997.4 ft./s2 is unassailable, and it does not matter whether the collision is elastic or inelastic. With a velocity reduction of 17.38 ft./s and a 997.4 ft./s2 deceleration, the duration of this impulse would have been 17 milliseconds. This rapid deceleration associated with the 31g impulse would necessarily show itself as an abrupt negative slope change in the velocity curve.
We have shown the curve starting upward again after the impact, on the generous assumption that the impacting object (the upper block) is now free to accelerate. We have also only charted what the effect on the velocity would have been for an initiating impulse between the first two floors to collide.
The measurements of the roof’s actual fall do not show any abrupt negative change in velocity, so it appears that there was no impulse and thus no amplified load. It seems that Dr. Bazant was simply theorizing that there had to be one to make sense of the collapse in a natural way. It is also important to note here that Dr. Bazant was off by a factor of ten in his calculation of the stiffness of the columns, with his 71 GN/m estimate. [8] The actual stiffness, calculated here using the actual column cross sections, is approximately 7.1 GN/m. (see Appendices B and C) [19][20] This error caused Dr. Bazant to significantly overestimate the potential amplifying effect of the impulse or jolt, which he claims occurred after a one story fall of the upper block.
In an effort to refute the argument put forth in this paper, some may claim that plastic deformation of the lower stories of the upper block could have created a crush wave below the upper block and kept the roof from experiencing a discernable impulse. If that were true then the impulse durations would have increased dramatically, absorbing the energy over a longer period of time and eliminating any significant amplification of the upper block’s weight. But without the amplification of the upper block’s weight why would the lower block have collapsed?
There are those who might argue that the tilt of the upper block to the south could have kept an impulse from being discernable—that there may have been impulses on the south face or further inside the Tower, in the central core, that were not visible on the north face. Impulses at these locations could not have caused the collapse of the north face of the Tower and its corner columns in the observed vertical manner. The corner columns of the east and west faces, in conjunction with the columns of the north face, formed a structural channel (a stiff structural element with support in two orthogonal directions) and, barring planned demolition, would have collapsed as observed only if they were struck impulsively, in a vertical manner by the upper block.
In reality, the upper block could not have tolerated the potential 31g impulse theorized by Dr. Bazant. To get this overload he claims was possible, all of the mass of the upper block would have had to participate, and if it did so it would have come apart completely.